Mathematics desk
< December 1	<< Nov \| December \| Jan >>	December 3 >

Welcome to the Wikipedia Mathematics Reference Desk Archives
The page you are currently viewing is an archive page. While you can leave answers for any questions shown below, please ask new questions on one of the current reference desk pages.

December 2[edit]

Proof for Internal/External Division of Line Segment[edit]

Hello. What is the proof for internal/external division of a line segment? $P(x,y)=\left({\frac {sx_{1}+rx_{2}}{r+s}},{\frac {sy_{1}+ry_{2}}{r+s}}\right)$ such that $A(x_{1},y_{1})$ and $B(x_{2},y_{2})$ are the first and second points respectively, $r$ is the length of the first division, $s$ is the length of the second division, and $P$ divides line $AB$ . Thanks in advance. --Mayfare 01:53, 2 December 2007 (UTC)[reply]

I'm not quite familiar with your notation and terminology, and you do not state what it is that needs to be proved, but here is my attempt at a sketch.

In general, a line in the Cartesian plane can be described by a linear equation of the form ax + by = c, where the coefficients a, b and c are real numbers, and a and b are not both zero. To find an equation for the line through two distinct points A with coordinates (x₁,y₁) and B with coordinates (x₂,y₂), we have to find expressions for a, b and c such that both points satisfy the equation. A solution is given by

a = −(y₁ − y₂), b = x₁ − x₂, c = x₁y₂ − x₂y₁.

A straightforward computation now establishes that the point P with coordinates given by the expression involving r and s lies on the line through A and B. By looking at the distances between P and these two points, we see that they are in the ratio of r to s, so if r + s is the distance between A and B, then P divides the line segment connecting A and B into subsegments of the required lengths. --Lambiam 06:09, 2 December 2007 (UTC)[reply]

Segment dissection[edit]

Is is possibe to dissect a segment into five equal parts under Euclidean rules? If so, how is it done? 72.197.202.36 02:35, 2 December 2007 (UTC)[reply]

Same way it is done with trisection. Start by taking your first line. In some arbitrary direction from one endpoint of that line, draw a small segment and multiply the length by five geometrically (easily done by just repeating the length with a compass). Now draw the base of the triangle formed by these two lines, and from each point of the 5x line, draw a line parallel to the base. Those lines will pentasect the original line by the fact that lines parallel to one side of a triangle divide the other sides in a fixed ratio. SamuelRiv 04:33, 2 December 2007 (UTC)[reply]

The usual term for division into two equal parts is bisection, while dissection is applied more to hapless frogs and such. I'd prefer the term quinquisect(ion) as the continuation of bisection, trisection and quadrisection, which have Latin number prefixes attached to a Latin-derived form. For Greek-derived terms, which do not suggest division in equal parts but more a distinction into categories, we have dichotomy, trichotomy, tetrachotomy, pentachotomy, ... --Lambiam 06:25, 2 December 2007 (UTC)[reply]

Dissection is a term sometimes used in geometry, but not quite for this. Algebraist 18:24, 2 December 2007 (UTC)[reply]

Consider a spherical frog. . . --Lambiam 20:33, 2 December 2007 (UTC)[reply]

Somebody prove Riemann already[edit]

COME ON PEOPLE I'M GETTING BORED HERE

its been too long

Also is it 100% certain that Poincare's Conjecture is proven thanks to Perelman's proof of Thurston's geometrization conjecture? Someone mentioned to me that the proof was still under review although that might be wrong. now talk —Preceding unsigned comment added by 81.153.211.188 (talk) 19:07, 2 December 2007 (UTC)[reply]

Among the Millennium Prize Problems the Riemann hypothesis was still completely open as of this morning. As to the Poincaré conjecture, all groups who have examined the proof and are competent to judge it, have independently found that it holds. As far as I've heard, the paper has not yet been formally published, a prerequisite for the Millenium Prize. I'm not even sure it has been submitted for publication. For a proof that difficult, there is always a remote possibility that someone notices a subtle but irrepairable gap even after publication; only additional complete and completely formal proof verification by computer – not likely to be attempted any time soon – could provide a major further boost to our confidence in the result. --Lambiam 20:50, 2 December 2007 (UTC)[reply]

Probablility Question - Help Me Win the Elks' Lodge Raffle![edit]

OK. At 2:00 PM, California time (3 hours from this post), I will be heading out with my family for an event at the Elks' Lodge called "Christmas Tree Lane." They take several Christmas trees (about a dozen) and decorate them with donated prizes from sponsors - gift cards for stores, gift certificates for travel, etc. The usual value of prizes on each tree is about $250, often higher. People attending the event buy raffle tickets and then place tickets in a box at the base of each tree. At the end of the event, each box is taken and a winner pulled out. The winner of each box wins the prizes on that tree, as well as tree and decorations.

I intend to buy $20 in tickets. The question is this: What is the best strategy for how to distribute the tickets amongst all the boxes (i.e. which individual raffles to enter)? I know there are lots of variables (the values of each tree, the number of entries for each raffle) but I'm trying to draw up a generic strategy. My aunt Sharrie always puts one ticket in each box, then the remainder in her favorite. I'm leaning more towards finding the least favorite tree (i.e. less attractive prizes/decorations, perhaps the one in the corner with less visibility, etc) and dumping the whole twenty in there in the hopes of a higher probability of winning.

I know it's short notice, but I'd really like to harness the power of the Internet here. Can anyone suggest a strategy? I will post my results tonight after the event.

Thanks,

--KNHaw ^(talk) 19:08, 2 December 2007 (UTC)[reply]

That's really short notice, man! I'll give some quick ideas.

The problem can be stated as Maximizing

\sum _{k=1}^{N}{\frac {y_{k}}{T_{k}}}V_{k}

subject to

\sum _{k=1}^{N}y_{k}=Y

...

...where

N is the number of trees
k=1...N indexes those trees
$y_{k}$ are the tickets you put in box [tree] k
$T_{k}$ are the total number of tickets you expect to be in box k

(you may think of it as

O_{k}+y_{k}

, where

O_{k}

are the tickets you expect all the people but you to put in that box).

$V_{k}$ is the value you asign to the kth tree. (You may notice I have taken expected utility for granted to state this problem).
Finally, Y is the total number of tickets you bought.

You may then proceed to find the first-order conditions for your problem. You might want to manage such assignment so that for every box j where you put tickets (i.e.

y_{j}\neq 0

), then it must be the case that

{\frac {O_{j}}{O_{j}^{2}+2Y_{j}O_{j}+Y_{j}^{2}}}V_{i}={\underset {k}{Max}}{\frac {O_{k}}{O_{k}^{2}+2Y_{k}O_{k}+Y_{k}^{2}}}V_{k}

You won't put a ticket in one box unless the benefit of doing so is at least equal to the gain of putting it in any other box.

If there are many tickets (the fraction of tickets you have is negligible) then you will put tickets in boxes for which the ratio

{\frac {V_{i}}{T_{i}}}

is the greatest.

Pallida Mors 19:57, 2 December 2007 (UTC)[reply]

As you may have noticed, I have assumed you can "estimate" the number of tickets the rest of the people will put in the boxes. That may have origin in some thumb rule

U(V_{i})

. But of course the analysis above can be extended to find Nash Equilibria and more complex equilibrium concepts, if you want to suppose that the other participants will behave according to some strategical motif. Pallida Mors 20:16, 2 December 2007 (UTC)[reply]

Results

Well, I found a tree that seemed to optimize

{V_{i}}

while keeping

{T_{i}}

under control. Specifically, a tree done up with donated restaurant gift cards that turned out to be valued at about $1000 retail (vs. $300 for the others). Also, it drew very little attention, the most popular trees being the ones stocked with liquor (we are talking about the Elks, after all). When the tickets were poured into the rotating drum for the drawing, I noted perhaps only 20% more than the average tree and substantially less than the most popular trees.

Alas, it was not to be. I placed 30 tickets in the box but there were easily 1000+ in the drum when the winning ticket was pulled. So, while I might have managed to optimize my chances and payoff, I still had only a 3% chance of winning. The woman who did win was quite delighted, though, and all the money went to a good cause (with this particular tree, the Muscular Dystrophy Association).

In any case, thanks for the help! --KNHaw ^(talk) 02:35, 3 December 2007 (UTC)[reply]

Your words are welcome, KNHaw! And your last remarks on the raffle are celebrated. Pallida Mors 10:08, 3 December 2007 (UTC)[reply]

Hopefully Easy Geometry Term Question...[edit]

What is the name or term for this situation: Two lines that are parallel, briefly come together to kiss or touch before veering off again, away from each other, never to meet again. It is some kind of non-Euclidean geometry thing maybe, or something I heard when someone was describing how to draw a Venn diagram? Or the orbits of planets and stars? I don't know, but it is driving me crazy. Thanks Saudade7 17:48, 2 December 2007 (UTC)[reply]

The usual definition of parallel lines in general two-dimensional geometries is that two lines are parallel if they do not intersect, so this phenomenon by definition cannot occur. Of course, it's possible for two such lines to meet 'at infinity': in hyperbolic geometry one calls lines 'parallel' if they meet only at infinity and 'ultraparallel' if they meet nowhere, not even an infinity. Other than that, you're going to have to come up with a new definition of 'parallel' to have parallel lines touching each other. Algebraist 18:20, 2 December 2007 (UTC)[reply]

Yeah, if two geodesics pass through a point and have the same tangent vector there, then they are the same geodesic. That holds in Riemannian geometry in general, which includes all the common non-Euclidean geometries. —Keenan Pepper 20:07, 2 December 2007 (UTC)[reply]

P.S. Why is this question green? —Keenan Pepper 20:07, 2 December 2007 (UTC)[reply]

You asked for the name or term. 'Two lines briefly come together to kiss or touch before veering off again, away from each other, never to meet again'. Well, it is called intersecting lines. You requested the lines to be parallel, but they are not parallel if they kiss or touch. Bo Jacoby 21:36, 2 December 2007 (UTC).[reply]

If you forget about straight lines and switch to general curves, then you may say they are osculating, which literally means "kissing". Confusing Manifestation(Say hi!) 21:43, 2 December 2007 (UTC)[reply]

Is there a mathematical term for the way these two blue lines touch (inside the red box)?

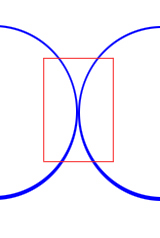
The question is green because I thought it was pretty. I know that in *our* space parallel lines don't touch, but I know that there are types of geometry where lines that we think of as parallel can touch, or even cross. Since I am not a mathematician I thought this happened in "non-Euclidean geometry" maybe. But I yield to the analytic point that "parallel" by definition means that the lines never touch. I think that maybe osculating *is* the term I was thinking of! I don't need it for anything mathy, I am just describing something in a painting. Keenan Pepper's answer, "if two geodesics pass through a point and have the same tangent vector there, then they are the same geodesic" was mind-boggling, reminding me both of a field trip in 3rd grade to an exhibit of Buckminster Fuller's works, and a B-grade sci-fi movie I once saw about space-lizards. I think Confusing Manifestation's answer about osculation is what I was thinking of, so he is a Mystic, for sure, given that I apparently didn't explain myself well. But MUCH THANKS TO EVERYONE!!! Saudade7 21:52, 2 December 2007 (UTC)

UPDATE: Maybe Unsolved???

To clarify: Is there a mathematical term for the way these two blue lines touch (inside the red box)? When I looked at osculating things they all seemed to be concentric like yolks in eggs)...Thanks for your help... Saudade7 22:39, 2 December 2007 (UTC)[reply]

For your picture it just means that the curves are tangent, a particular form of Contact (mathematics), as the curvatures are opposites in sign they are not actually osculating. --Salix alba (talk) 23:13, 2 December 2007 (UTC)[reply]

Thank you Salix alba. Saudade7 01:24, 3 December 2007 (UTC)[reply]

Tangent is certainly the most correct term, but if you just called those curves 'touching' I think you'd be understood. Note that to a mathematician, a line is not what you might think it is: those blue things are not lines, but curves. Algebraist 01:59, 3 December 2007 (UTC)[reply]

Merci beaucoup Algebraist, Alas, I just wanted a cool term. "Touching" is so quotidian! I really remember hearing an actual word once. I am thinking maybe it was in a paper presented by an analytic philosopher called "Can things touch?" Apparently the answer was "No." Oh well. Thanks for your help! Saudade7 02:33, 3 December 2007 (UTC)[reply]

If you're after a cool term (and don't mind being wildly imprecise), just call the point where the curves touch a singularity. That word means all kinds of things, and I'm sure it's because it sounds good. Algebraist 03:36, 3 December 2007 (UTC)[reply]

You can get precise with the singularities, if you define the curve as a the zero set of a map from R² to R, then the you have an example of an A₃ singularity at the origin, which has a normal form

x^{2}\pm y^{4}

. The picture also has additional symmetry and there are ways this can be captured in singularity theory by considering Bruce's fold maps. --Salix alba (talk) 12:45, 3 December 2007 (UTC)[reply]

Just to make life a little more complicated, there are situations where you could refer to the blue curves as lines (or line segments). For instance, I've seen it done when describing circles in the Poincare half-plane model, which these could very well be. Although I suppose the term geodesic would probably be more appropriate... 134.173.93.150 04:07, 3 December 2007 (UTC)[reply]

No, the two tangent circles could not be lines, even in the Poincaré half plane. In the Poincaré half plane, hyperbolic lines correspond to semicircles centered on the horizon line. Other circles not only appear curved; they actually are curved in hyperbolic geometry, i.e., they're not geodesics. Two geodesics cannot be tangent in any Riemannian manifold, as I said before. —Keenan Pepper 05:32, 3 December 2007 (UTC)[reply]

A point of osculation is known as a tacnode 86.146.175.166 20:40, 3 December 2007 (UTC)[reply]