User:Tomruen/Rectified orthoplex
Appearance
Quick rebuild from orthoplex.
The class of rectified orthoplexes are special for having a circumradius equal to their edge-length. Thus they all can be used as vertex figures for uniform tessellations. Tom Ruen (talk) 21:54, 1 June 2010 (UTC)
| n | Name(s) Graph |
Graph 2n-gon |
Schläfli | Coxeter-Dynkin diagrams |
Vertices | Edges | Faces | Cells | 4-faces | 5-faces | 6-faces | 7-faces | 8-faces | 9-faces |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | Bicross square rectified 2-orthoplex |
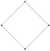
|
t1{4} | 4 | 4 | |||||||||
| 3 | Tricross cuboctahedron rectified 3-orthoplex |

|
t1{3,4} | 12 | 24 | 14 | ||||||||
| 4 | rectified tetracross 24-cell rectified 4-orthoplex |

|
t1{3,3,4} t1{31,1,1} |
24 | 96 | 96 | 24 | |||||||
| 5 | Rectified pentacross rectified 5-orthoplex |

|
t1{33,4} t1{32,1,1} |
40 | 240 | 400 | 240 | 42 | ||||||
| 6 | rectified hexacross rectified 6-orthoplex |

|
t1{34,4} t1{33,1,1} |
60 | 480 | 1120 | 1200 | 576 | 76 | |||||
| 7 | rectified heptacross rectified 7-orthoplex |

|
t1{35,4} t1{34,1,1} |
84 | 840 | 2520 | 3920 | 3360 | 1344 | 142 | ||||
| 8 | rectified octacross rectified 8-orthoplex |

|
t1{36,4} t1{35,1,1} |
112 | 1344 | 4928 | 10080 | 12544 | 8960 | 3072 | 272 | |||
| 9 | rectified enneacross rectified 9-orthoplex |

|
t1{37,4} t1{36,1,1} |
|||||||||||
| 10 | rectified decacross rectified 10-orthoplex |

|
t1{38,4} t1{37,1,1} |
