User:Wagnertb/sandbox

O efeito Aharonov–Bohm, algumas vezes chamado de efeitoEhrenberg–Siday–Aharonov–Bohm, é um fenômeno quântico no qual uma partícula pontual eletricamente carregada é afetada por um campo eletromagnético (E, B), apesar de estar confinada numa área na qual ambos campo magnético B and campo elétrico E são zero. O mecânismo principal para isso ocorra é o acoplamento do potencial eletromagnético com a fase complexa da função de onda de uma partícula carregada , e o efeito Aharonov–Bohm é coerentemente ilustrado pelo experimento da fenda dupla.

O caso mais comum, chamado de efeito Aharonov–Bohm no solenóide , acontece quando a função de onda de uma partícula carregada que passa ao redor de um solenóide longo sofre uma mudança de fase causada pelo campo magnético contido dentro do solenóide, apesar do campo ser despresível na região na qual a partícula passa e a função de onda da partícula ser desprezível dentro do solenoide. Essa mudança de fase foi observada experimentalmente. Existem também efeitos Aharonov–Bohm magnéticos

em energias ligadas e seções de choque de espalhamento, mas esses caso não foram testados experimentalmente. Um fenômeno Aharonov–Bohm elétrico foi também previsto, no qual uma partícula carregada é afetada por regiões com diferentes potenciais elétricos mas campo elétrico nulo, embora não haja confirmação experimental ainda. Um tipo de efeito Aharonov–Bohm "molecular" foi proposto para o movimento nuclear em regiões multiplamente conectadas, mas ele foi tido como sendo um tipo diferente de fase geométrica porque não é "nem não-local nem topológico", dependendo apenas de quantidades locais ao longo do caminho nuclear.

Werner Ehrenberg e Raymond E. Siday previram o efeito primeiro em 1949, e efeitos similares foram depois publicados por Yakir Aharonov e David Bohm em 1959. Depois da publicação do artigo de 1959 , Bohm foi informado do trabalho de Ehrenberg e Siday, o qual reconheceu e foi creditado no artigo subsequente de Bohm e Aharonov em 1961. Posteriormente o efeito foi verificado experimentalmente por vários autores; uma revisão geral pode ser encontrada em Peshkin e Tonomura (1989).

Significância

Nos séculos 18 e 19, a física era dominada pela dinâmica Newtoniana, com ênfase nas forças. Fenômenos eletromagnéticos elucidados por uma série de experimentos envolvendo medidas de forças entre cargas, correntes elétricas e ímãs em várias configurações. Eventualmente, apareceu uma descrição de acordo com como cada carga, corrente e imã age como uma fonte local de campos de forças propagantes, os quais então atua em outras cargas e correntes localmente através da força de Lorentz. Nessa situação, porque uma das propriedades observadas do campo elétrico é que ele é irrotational, e uma das propriedades observadas do campo magnético é que ele é não-divergente, foi possível expressar o campo eletrostático como o gradiente de um potencial escalar(o potencial eletrostático deCoulomb, inteiramente análogo, matematicamente, ao potencial gravitacional clássico) e o campo magnético estacionário como o rotacional de um campo vetorial (um novo conceito pra época – a ideia de um potencial escalar já era bem aceita pela analogia com o potencial gravitacional). A linguagem de potenciais foi generalizada para o caso completamente dinâmico mas, como todos os efeitos físicos foram descritos em termos dos campos, que são derivadas dos potenciais, os potenciais (diferentemente dos campos) não foram unicamente determinados pelos experimentos físicos: Potenciais foram definidos apenas até um potencial constante aditivo não-divergente e um e um potencial vetorial magnético constante irrotacional.

O efeito Aharonov–Bohmé importante conceitualmente porque consiste em três problemas que aparecem na teoria de James Clerk Maxwel sobre o eletromagnetismo clássico como uma teoria de calibre, a qual antes do advento da mecânica quântica era tratado como uma reformulação matemática sem consequências físicas. O experimento mental (Gedanken) de Aharonov–Bohm e sua realização experimental implica que os problemas não eram só filosóficos.

Os três problemas são:

1. Se os potenciais são "físicos" ou só uma ferramenta conveniente para calcular campos de força;
2. Se o princípio da mínima ação é fundamental;
3. O princípio da localidade.

Por razões como essa, o efeito Aharonov–Bohm foi escolhido pela revista New Scientist como uma das "sete maravilhas do mundo quântico".

Potenciais vs Campos

É comumente argumentado que o efeito Aharonov–Bohm ilustra a fisicalidade dos potenciais eletromagnéticos,Φ e A, na mecânica quântica. Classicamente foi possível dizer que apenas os campos eletromagnéticos são físicos, enquanto que os potenciais são construções puramente matemáticas, devido à liberdade de calibre não ser nem única, para um dado campo eletromagnético.

Entretanto, Vaidman desafiou essa interpretação, mostrando que o efeito AB pode ser explico sem o uso de potenciais contanto que se dê um tratamento puramente quântico para as cargas que produzem o campo eletromagnético. De acordo com esse ponto de vista, o potencial na mecânica quântica é tão físico quanto é classicamente.

Ação Global vs. Forças Locais

Similarmente, o efeito Aharonov–Bohm ilustra que a abordagem Lagrangiana à dinâmica, baseada em energias, não é apenas uma ferramenta computacional para a abordagem Newtoniana, baseada em forças. Contudo, o efeito Aharonov–Bohm valida o ponto de vista de que forças são um forma incompleta de formular a física, e que energias potenciais devem ser usadas ao invés. De fato, pt:Richard Feynman já havia reclamado que a ele foi ensino o eletromagnetismo do ponto de visto dos campos elétricos, e ele desejou mais tarde em sua vida que tivesse sido ensinado a ele a pensar em termos dos potenciais eletromagnéticos, pois é uma abordagem mais fundamental. Na formulação das integrais de caminho de Feynman, o campo potencial muda diretamente a fase da função de onda de um elétron e essas mudanças de fase que levam à quantidades mensuráveis.

Localidade dos campos eletromagnéticos

O efeito Aharonov–Bohm mostra que os campos locais E e B não contém informação completa sobre o campo eletromagnético e que o potencial quadridimensional (Φ,A) deve ser usado no lugar. Pelo teorema de Stokes, a magnitude do efeito AB pode ser calculada usando somente os campos eletromagnéticos, ou usando o potencial quadridimensional ao invés. Mas quando usados os campos eletromagnéticos, o efeito depende do valor do campo em uma região na qual as partículas de teste são proibidas. Em contraste, quando usado o potencial quadridimensional, o efeito depende apenas no potencial na região na qual as partículas de teste são permitidas. Com isso, uma pessoa pode ou abandonar o princípio da localidade, o que muitos físicos são relutantes sobre fazer, ou ser forçada a aceitar que o potencial quadridimensional eletromagnético oferece uma descrição mais completa do eletromagnetismo que os campos elétricos e magnéticos podem. Pelo outro lado, o efeito AB é crucialmente quântico; mecânica quântica é bem conhecida por apresentar efeitos não-locais, e Vaidman argumentou que esse é apenas um efeito quântico não-local em uma forma diferente.

No eletromagnetismo clássico as duas descrições são equivalente. Com a adição da teoria quântica, porém, os potenciais eletromagnéticos são vistos como mais fundamentais.  Apesar disso, todos os efeitos observáveis acabam sendo expressáveis em termos dos campos eletromagnéticos E e B. Isso é interessante porque, mesmo que se possa calcular os campos eletromagnéticos do vetor quadridimensional, devido à liberdade de calibre, o inverso não é verdadeiro.

Efeito no solenoide magnético

O efeito Aharonov–Bohm pode ser visto como um resultado da necessidade da mecanica quântica de ser invariante com respeito à escolha de calibre para o potencial eletromagnético de qual faz parte o vetor potencial Magnético A.

A teoria eletromagnetica implica que uma partícula com carga elétrica q viajando ao longo de um caminho P em uma região com campo magnético nulo, mas potencial A não-nulo, adquite uma mudança de fase $\varphi$, dada em unidades do SI por

$\varphi ={\frac {q}{\hbar }}\int _{P}\mathbf {A} \cdot d\mathbf {x} ,$

Portanto, partículas, com os mesmos pontos de partida e chegada, mas que viajam através de duas rotas diferentes vão adquiri uma fase diferente $\Delta \varphi$ determinada pelo fluxo magnético$\Phi _{B}$ através da área entre os caminho (pelo teorema de Stokes e $\nabla \times \mathbf {A} =\mathbf {B}$), dado por:

$\Delta \varphi ={\frac {q\Phi _{B}}{\hbar }}.$

Esquema of experimento da fenda dupla na qual o efeito AB pode ser observado: eléctrons passam através duas fendas, interferindo em uma tela de observação, com os padrões de interferência deslocados quando um campo magnético B é ligado no solenoide cilíndrico.

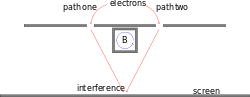
Na mecânica quântica a mesma partícula pode viajar entre dois pontos por uma variedade de caminho. Por isso, essa diferença de fase pode ser observada se colocado um solenóide entre as fendas de um experimento de fenda dupla (ou equivalente). Um solenoide ideal (i.e. infinitamente longo e com uma distribuição perfeitamente uniforme) engloba o campo magnético B, mas não produz nenhum campo eletromagnético fora de seu cilindro, e então a partícula (e.g. um elétron) passando por fora não sente nenhum campo magnético B. Entretanto, existe um potencial vetor de rotacional nulo A fora do solenoide com um fluxo englobado. Então a fase relativa das partículas passando através de uma fenda ou de outra é alterada por se a corrente do solenoide está ligada ou desligada. Isso corresponde à mudança das franja de interferência no plano de observação.

O mesmo efeito na fase é responsável pela necessidade de quantização do fluxo em circuitos fechados supercondutores. Esse quantização ocorre porque a função de onda supercondutora deve ter valor únicdo em todos os ponto: sua diferença de fase $\Delta \varphi$ em torno de um circuito fechado deve ser um múltiplo inteiro de 2π (com a carga sendo q=2e para o par de elétrons de Cooper), e por isso o fluxo deve ser um múltiplo de h/2e. O quanta de fluxo supercondutor foi na verdade previsto anteriormente ao efeito AB, por F. Londo em 1948 usando um modelo fenomenológico.

A primeira afirmação de confirmação experimental foi feito por sadasdasdsad em 1968, em um interferômetro de elétrons com um campo magnético produzido por um fio de ferro, e outro trabalho é resumido em Olariu e Papêscu (1984). Entretando, autores subsequentes questionaram a validade de vários destes resultados, porque os elétrons podem não ter sido completamente blindados dos campos magnéticos. Um experimento no qual um efeito AB não ambíguo foi observado através da completa exclusão do campo magnético do caminho do elétron (com a ajuda de um filme supercondutor) foi feito por Tonomura et al. em 1986. O escopo do efeito e suas aplicações continuam a se expandir. Webb et all. (1985) demonstraram oscilações AB em anéis metálicos ordinários, não-supercondutores; para uma discussão, veja Schwarzschild (1986) e Imry & Webb (1989). Bachtold et al. (1999) detectou o efeito em nanotubos de carbono; para uma discussão, veja Kong et al. (2004).

Monopoles and Dirac strings

The magnetic Aharonov–Bohm effect is also closely related to Dirac's argument that the existence of a magnetic monopole can be accommodated by the existing magnetic source-free Maxwell's equations if both electric and magnetic charges are quantized.

A magnetic monopole implies a mathematical singularity in the vector potential, which can be expressed as a Dirac string of infinitesimal diameter that contains the equivalent of all of the 4πg flux from a monopole "charge" g. The Dirac string starts from, and terminates on, a magnetic monopole. Thus, assuming the absence of an infinite-range scattering effect by this arbitrary choice of singularity, the requirement of single-valued wave functions (as above) necessitates charge-quantization. That is, $2{\frac {q_{\text{e}}q_{\text{m}}}{\hbar c}}$ must be an integer (in cgs units) for any electric charge q_e and magnetic charge q_m.

Like the electromagnetic potential A the Dirac string is not gauge invariant (it moves around with fixed endpoints under a gauge transformation) and so is also not directly measurable.

Electric effect

Just as the phase of the wave function depends upon the magnetic vector potential, it also depends upon the scalar electric potential. By constructing a situation in which the electrostatic potential varies for two paths of a particle, through regions of zero electric field, an observable Aharonov–Bohm interference phenomenon from the phase shift has been predicted; again, the absence of an electric field means that, classically, there would be no effect.

From the Schrödinger equation, the phase of an eigenfunction with energy E goes as $\exp(-iEt/\hbar )$. The energy, however, will depend upon the electrostatic potential V for a particle with charge q. In particular, for a region with constant potential V (zero field), the electric potential energy qV is simply added to E, resulting in a phase shift:

$\Delta \phi =-{\frac {qVt}{\hbar }},$

where t is the time spent in the potential.

The initial theoretical proposal for this effect suggested an experiment where charges pass through conducting cylinders along two paths, which shield the particles from external electric fields in the regions where they travel, but still allow a varying potential to be applied by charging the cylinders. This proved difficult to realize, however. Instead, a different experiment was proposed involving a ring geometry interrupted by tunnel barriers, with a bias voltage V relating the potentials of the two halves of the ring. This situation results in an Aharonov–Bohm phase shift as above, and was observed experimentally in 1998.

Aharonov–Bohm nano rings

Nano rings were created by accident while intending to make quantum dots. They have interesting optical properties associated with excitons and the Aharonov–Bohm effect. Application of these rings used as light capacitors or buffers includes photonic computing and communications technology. Analysis and measurement of geometric phases in mesoscopic rings is ongoing.

Several experiments, including some reported in 2012, show Aharonov-Bohm oscillations in charge density wave (CDW) current versus magnetic flux, of dominant period h/2e through CDW rings up to 85 µm in circumference above 77 K. This behavior is similar to that of the superconducting quantum interference devices (see SQUID).

Mathematical interpretation

The Aharonov–Bohm effect can be understood from the fact that we can only measure absolute values of the wave function. While this allows us to measure phase differences through quantum interference experiments, we have no way to specify a wavefunction with constant absolute phase. In the absence of an electromagnetic field we can come close by declaring the eigenfunction of the momentum operator with zero momentum to be the function "1" (ignoring normalization problems) and specifying wave functions relative to this eigenfunction "1". In this representation the i-momentum operator is (up to a factor $\hbar /i$) the differential operator $\partial _{i}={\frac {\partial }{\partial x^{i}}}$. However, by gauge invariance, it is equally valid to declare the zero momentum eigenfunction to be $e^{-i\phi (x)}$ at the cost of representing the i-momentum operator (up to a factor) as $\nabla _{i}=\partial _{i}+i(\partial _{i}\phi )$ i.e. with a pure gauge vector potential $A=d\phi$. There is no real asymmetry because representing the former in terms of the latter is just as messy as representing the latter in terms of the former. This means that it is physically more natural to describe wave "functions", in the language of differential geometry, as sections in a complex line bundle with a hermitian metric and a U(1)-connection $\nabla$. The curvature form of the connection, $iF=\nabla \wedge \nabla$, is, up to the factor i, the Faraday tensor of the electromagnetic field strength. The Aharanov–Bohm effect is then a manifestation of the fact that a connection with zero curvature (i.e. flat), need not be trivial since it can have monodromy along a topologically nontrivial path fully contained in the zero curvature (i.e. field free) region. By definition this means that sections that are parallelly translated along a topologically non trivial path pick up a phase, so that covariant constant sections cannot be defined over the whole field free region.

Given a trivialization of the line-bundle, a non-vanishing section, the U(1)-connection is given by the 1-form corresponding to the electromagnetic four-potential A as $\nabla =d+iA\,$ where d means exterior derivation on the Minkowski space. The monodromy is the holonomy of the flat connection. The holonomy of a connection, flat or non flat, around a closed loop $\gamma$ is $e^{i\int _{\gamma }A}$ (one can show this does not depend on the trivialization but only on the connection). For a flat connection we can find a gauge transformation in any simply connected field free region(acting on wave functions and connections) that gauges away the vector potential. However, if the monodromy is nontrivial, there is no such gauge transformation for the whole outside region. In fact as a consequence of Stokes' theorem, the holonomy is determined by the magnetic flux through a surface $\sigma$ bounding the loop $\gamma$, but such a surface may exist only if $\sigma$ passes through a region of non trivial field:

$e^{i\int _{\partial \sigma }A}=e^{i\int _{\sigma }dA}=e^{i\int _{\sigma }F}$

The monodromy of the flat connection only depends on the topological type of the loop in the field free region (in fact on the loops homology class). The holonomy description is general, however, and works inside as well as outside the superconductor. Outside of the conducting tube containing the magnetic field, the field strength $F=0$. In other words, outside the tube the connection is flat, and the monodromy of the loop contained in the field-free region depends only on the winding number around the tube. The monodromy of the connection for a loop going round once (winding number 1) is the phase difference of a particle interfering by propagating left and right of the superconducting tube containing the magnetic field. If we want to ignore the physics inside the superconductor and only describe the physics in the outside region, it becomes natural and mathematically convenient to describe the quantum electron by a section in a complex line bundle with an "external" flat connection $\nabla$ with monodromy

$\alpha =$ magnetic flux through the tube / $(\hbar /e)$

rather than an external EM field $F$. The Schrödinger equation readily generalizes to this situation by using the Laplacian of the connection for the (free) Hamiltonian

$H={\frac {1}{2m}}\nabla ^{*}\nabla$.

Equivalently, we can work in two simply connected regions with cuts that pass from the tube towards or away from the detection screen. In each of these regions we have to solve the ordinary free Schrödinger equations but in passing from one region to the other, in only one of the two connected components of the intersection (effectively in only one of the slits) we pick up a monodromy factor $e^{i\alpha }$, which results in the shift in the interference pattern as we change the flux.

Effects with similar mathematical interpretation can be found in other fields. For example, in classical statistical physics, quantization of a molecular motor motion in a stochastic environment can be interpreted as an Aharonov–Bohm effect induced by a gauge field acting in the space of control parameters.

See also

• Geometric phase
• Hannay angle
• Wannier function
• Berry phase
• Wilson loop
• Winding number
• Aharonov–Casher effect
• Byers-Yang theorem

General references

• D. J. Thouless (1998). "§2.2 Gauge invariance and the Aharonov–Bohm effect". Topological quantum numbers in nonrelativistic physics. World Scientific. pp. 18ff. ISBN 981-02-3025-7.

External links

• The David Bohm Society page about the Aharonov–Bohm effect.
• A video explaining the use of the Aharonov–Bohm effect in nano-rings.

Category:Quantum mechanics Category:Physical phenomena Category:Mesoscopic physics